Tim Chartier, a math professor at Davidson College, found a way to express a principle of calculus using the best of all possible source materials: chocolate. He created a series of enlarging charts featuring a growing number of chocolate chips:
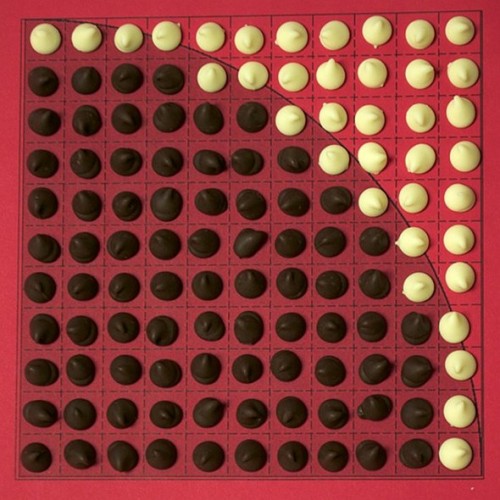
If you count carefully, we use 83 milk chocolate chips of the 121 total. This gives us an estimate of 2.7438 for ?, which correlates to an error of about 0.378. [...]
What do you notice is happening to the error as we reduce the size of the squares? Indeed, our estimates are converging to the exact area. Here lies a fundamental concept of Calculus. If we were able to construct such chocolate chip mosaics with grids of ever increasing size, then we would converge to the exact area. Said another way, as the area of the squares approaches zero, the limit of our estimates will converge to ?. Keep in mind, we would need an infinite number of chocolate chips to estimate ? exactly, which is a very irrational thing to do!

No comments:
Post a Comment